Question

Determine the number of real solutions for each of the given equations. Equation Number of Solutions y = -3x2 + x + 12 y = 2x2 - 6x + 5 y = x2 + 7x - 11 y = -x2 - 8x - 16 R

Answer 1

The first box is "two real solutions," the second box is "no real solution," the third box is "two real solution," and the fourth box is "one real solution".

Explanation:

Answer 2

Explanation:

Our equations are

`y = -3x^2 + x + 12\\y = 2x^2 - 6x + 5\\y = x^2 + 7x - 11\\y = -x^2 - 8x - 16\\`

Let us understand the term Discriminant of a quadratic equation and its properties

Discriminant is denoted by D and its formula is

`D=b^2-4ac\\`

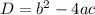
Where

a= the coefficient of the
`x^(2)`

b= the coefficient of
`x`

c = constant term

Properties of D: If D

i) D=0 , One real root

ii) D>0 , Two real roots

iii) D<0 , no real root

Hence in the given quadratic equations , we will find the values of D Discriminant and evaluate our answer accordingly .

Let us start with

`y = -3x^2 + x + 12\\a=-3 , b =1 , c =12\\D=1^2-4*(-3)*(12)\\D=1+144\\D=145\\D>0 \\`

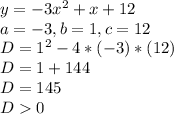
Hence we have two real roots for this equation.

`y = 2x^2 - 6x + 5\\`

`y = 2x^2 - 6x + 5\\a=2,b=-6,c=5\\D=(-6)^2-4*2*5\\D=36-40\\D=-4\\D<0\\`

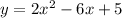
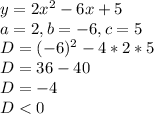
Hence we do not have any real root for this quadratic

`y = x^2 + 7x - 11\\a=1,b=7,-11\\D=7^2-4*1*(-11)\\D=49+44\\D=93\\`

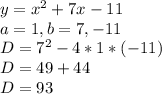
Hence D>0 and thus we have two real roots for this equation.

`y = -x^2 - 8x - 16\\a=-1,b=-8,c=-16\\D=(-8)^2-4*(-1)*(-16)\\D=64-64\\D=0\\`

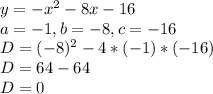
Hence we have one real root to this quadratic equation.