Question

1. An angle in a right triangle is identified as θ. If the tangent of θ equals one, what must be true about the triangle side lengths?

A. The side adjacent to theta is half the length of the hypotenuse.
B. The side opposite to theta is longer than the adjacent side.
C. The sides opposite and adjacent to theta are the same length.
D. The side adjacent to theta is longer than the adjacent side.

Answer 1

C. The sides opposite and adjacent to theta are the same length.

Explanation:

Given : tanθ = 1

recall tanθ =
`(opposite)/(adjacent)`

the only way for
`(opposite)/(adjacent)` to equal 1, is that the numerator is the same value as the denominator,

hence the answer is

C. The sides opposite and adjacent to theta are the same length.

Answer 2

Answer: Option C

"The sides opposite and adjacent to theta are the same length."

Explanation:

By definition the tangent of an angle
`\theta` is written as:

`tan(\theta) = (opposite)/(adjacent)`

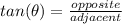
Where:

"opposite" is the side opposite the
`\theta` angle

"adjacent" is the side that contains the angle
`\theta` and the angle of 90 °.

In this case we know that

`tan(\theta) = (opposite)/(adjacent) = 1`

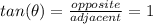
If
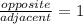`(opposite)/(adjacent) = 1` then
`opposite = adjacent`

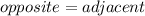
Finally the answer is the option C

"The sides opposite and adjacent to theta are the same length."