Answer:
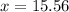
Explanation:
We can use the law of sines here. However, we will first need to solve for the missing angle.
The interior angles of a triangle add up to 180.
Therefore,
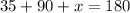
where
 is the missing angle.
is the missing angle.
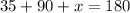

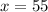
So the missing angle is 55 degrees.
Now we can use the law of sines to set up a proportion.
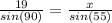
Now simplify the equation.
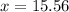
Rounded to the nearest hundredth.