Answer: The required solution of the given quadratic equation is
x = 3 + √3 and x = 3 - √3.
Step-by-step explanation: We are given to solve the following quadratic equation using quadratic formula :

Quadratic formula : The solution of a quadratic equation of the form
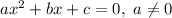 is given by
is given by
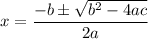
For the given quadratic equation (i), we have
a = 1, b = -6 and c = 6.
Therefore, the solution of equation (i) is given by
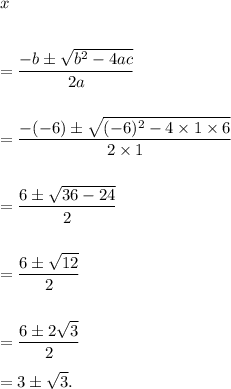 .
.
Thus, the required solution of the given quadratic equation is
x = 3 + √3 and x = 3 - √3.