Question

How do you: Find the 4th term of (x-2y)^6

Answer 1

The fourth term is
`-160x^(3)y^3`.

Explanation:

The given binomial expression is
`(x-2y)^6`.

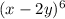When we compare this to the general binomial expression,
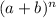`(a+b)^n`, we have
`a=x,b=-2y,n=6`.

The specific term in a binomial expansion with an integral index is given by:

`T_(r+1)=\binom{n}{r}a^(n-r)b^r\:\:or\:\:T_(r+1)=^nC_ra^(n-r)b^r`.

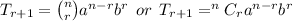To find the fourth term, we set
`r+1=4`. This implies that;
`r=4-1=3`.

We now substitute the values into the formula to obtain:

`T_(3+1)=\binom{6}{3}x^(6-3)(-2y)^3`.

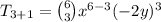We simplify to get:

`T_(4)=20x^(3)(-8y^3)`.

`T_(4)=-160x^(3)y^3`.

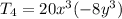Therefore the fourth term is
`-160x^(3)y^3`.