Question

Jillian is trying to save water, so she reduces the size of her square grass lawn by 8 feet on each side. The area of the smaller lawn is 144 square feet. In the equation

(x – 8)2 = 144, x represents the side measure of the original lawn.

What were the dimensions of the original lawn?

4 feet by 4 feet
8 + feet by 8 +
8 feet by 8 +
20 feet by 20 feet

Answer 1

20 by 20

Explanation:

the new dimensions have to be 12 because 12×12 =144 so you would add 8 to 12 and get 20 for each side

Answer 2

D) 20 feet by 20 feet

Explanation:

Given:

The area of the original grass lawn reduced by 8 feet on each side.

The smaller lawn's area = 144 square feet which is represented by the equation

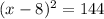`(x - 8)^2 = 144`, where "x" is the side of the original lawn.

To find the original dimension of the lawn, we need to solve for x from the above equation.

To solve follow the steps.

Step 1:

To get rid of square on the right hand side, we need to take square root on both sides.

Taking the square root on both sides, we get

`√((x - 8)^2) = √(144)`

`(x - 8) = 12`

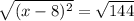
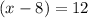
Step 2:

Now add 8 on both sides, we get

x - 8 + 8 = 12 + 8

x = 20

Therefore, the original dimensions of the lawn is 20 feet by 20 feet.