Question

????????????????????????

Answer 1

C. Center (3,-2) and radius 3

Explanation:

The given circle has equation:

`x^2+y^2-6x+4y+4=0`

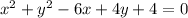
We rearrange to get:

`x^2-6x+y^2+4y=-4`

We add the square of half the coefficients of the linear terms to both sides of the equation:

`x^2-6x+(-3)^2+y^2+4y+(2)^2=-4+(-3)^2+(2)^2`

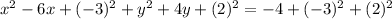
We factor the perfect squares and simplify to get;

`(x-3)^2+(y+2)^2=9`

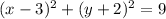
We can rewrite as;

`(x-3)^2+(y--2)^2=3^2`

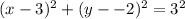
Comparing this to the standard equation of the circle;

`(x-h)^2+(y-k)^2==r^2`

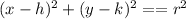
We have (3,-2) and the center and r=3 as the radius.