Question

Liam has to fill a hole in the ground that is 24 inches deep. He fills the hole at a rate of 6 inches in 30 minutes. Write a function that models the depth of the hole in feet over time in hours.

Answer 1

The function that models the depth of the hole in feet over time in hours is
`h(t) = 24 - 12\cdot t`.

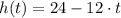Explanation:

According to the statement, the variable to be modelled is the depth of the hole, which decreases whereas is filled. Under the assumption that the hole is filled at constant rate, we obtain the following expression:

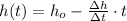`h(t) = h_(o)-(\Delta h)/(\Delta t)\cdot t` (1)

Where:

`h(t)` - Current depth of the hole, measured in inches.

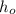`h_(o)` - Initial depth of the hole, measured in inches.

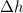`\Delta h` - Filled level, measured in inches.

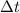`\Delta t` - Filling time, measured in hours.

`t` - Time, measured in hours.

If we know that
`h_(o) = 24\,in`,
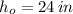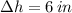`\Delta h = 6\,in` and
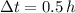`\Delta t = 0.5\,h`, then the function that models the depth of the hole is:

`h(t) = 24 - 12\cdot t`

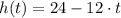
The function that models the depth of the hole in feet over time in hours is
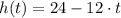`h(t) = 24 - 12\cdot t`.