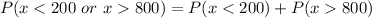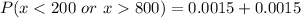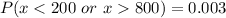Answer:
The probability is 0.003
Explanation:
We know that the average
 is:
is:

The standard deviation
 is:
is:
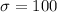
The Z-score is:
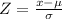
We seek to find
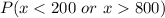
For P(x>800) The Z-score is:
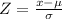
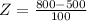
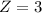
The score of Z = 3 means that 800 is 3 standard deviations from the mean. Then by the rule of the 8 parts of the normal curve, the area that satisfies the conficion of 3 deviations from the mean has percentage of 0.15%
So

For P(x<200) The Z-score is:
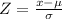
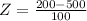

The score of Z = -3 means that 200 is 3 standard deviations from the mean. Then by the rule of the 8 parts of the normal curve, the area that satisfies the conficion of 3 deviations from the mean has percentage of 0.15%
So

Therefore