Answer:
a) f(x) is a homogenous function of degree '1'
b) f(x) is a homogenous function of degree '2'
Explanation:
Step(i):-
Homogenous function
If f(x) is a homogenous function of degree 'n' then
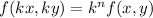
a)
Given
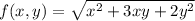
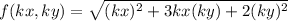
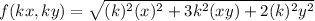

f( k x , k y ) = k f( x, y)
∴ f(x) is a homogenous function of degree '1'
Step(ii):-
b)

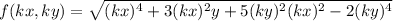

f(k x , k y ) = k² f( x , y )
∴ f(x) is a homogenous function of degree '2'