Answer:
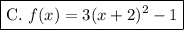
Explanation:
The vertex form of a quadratic function
ƒ(x) = a(x - h)² + k, where (h, k) is the vertex of the parabola.
You convert ƒ(x) = 3x² + 12x + 11 to the vertex form by completing the square.
Step 1. Move the constant term to the other side of the equation
y - 11 =3x² + 12x
Step 2. Factor out the leading coefficient
y - 11 =3(x² + 4x)
Step 3. Complete the square on the right-hand side
Take half the coefficient of x, square it, and add it to each side of the equation.
4/2 = 2; 2² = 4
y – 11 + 12 =3(x² + 4x + 4)
Note that when you completed the square by adding 4 inside the parentheses, you were adding 3×4 to the right-hand side, so you had to add 12 to the left-hand side.
Step 4. Simplify and write the right-hand side as a perfect square
y + 1 = 3(x + 2)²
Step 5. Isolate the y term
Subtract 1 from each side
y = 3(x + 2)² -1

If you compare this equation with the general vertex form and with the graph, you will find that h = -2 and k = -1, so the vertex is at (-2, -1).