Question

PLEASE HELP!!

A cat is watching a bird in a tree nearby. The tree is approximately 20 ft from the cat (ground distance). If the cat’s line of sight makes a 25° with the ground when he has his eye on the bird, how high up is the bird in the tree?

A. Draw a picture


B. Solve the problem, Solve to the nearest ft.

Answer 1

The bird is approximately 9 ft high up in the tree

Step-by-step explanation:

The required diagram is shown in the attached image

Note that the tree, the cat and the ground form a right-angled triangle

Therefore, we can apply special trigonometric functions

These functions are as follows:

`sin(\alpha)=(opposite)/(hypotenuse) \\ \\ cos(\alpha)=(adjacent)/(hypotenuse) \\ \\tan(\alpha)=(opposite)/(adjacent)`

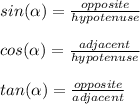
Now, taking a look at our diagram, we can note the following:

α = 25°

The opposite side is the required height (x)

The adjacent side is the distance between the cat and the tree = 20 ft

Therefore, we can use the tan function

This is done as follows:

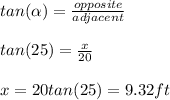`tan(\alpha)=(opposite)/(adjacent)\\ \\ tan(25)=(x)/(20)\\ \\x=20tan(25) = 9. 32 ft` which is 9 ft approximated to the nearest ft

Hope this helps :)