Question

Line segment XY is a directed line segment beginning at point X(9, 2) and ending at point Y(-6, 2). Find the point Z on the line segment that partitions the line segment into the segments XZ and ZY at a ratio of 5:3.

Answer 1

`(-(3)/(8),2)`

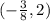
Explanation:

Point Z divides XY into a 5:3 ratio, so Z is 5/3 of the way from X to Y. That ratio is k, found by writing the numerator of the ratio (5) over the sum of the numerator and the denominator (5 + 3 = 8). Our k value is 5/8. Now we will find the rise and run values which is the slope of this line segment:

`m=(2-2)/(-6-9) =(0)/(-15)`

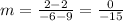
Coordinates are found in this formula:

`Z(x,y)=(x_(1)+k(run),y_(1) +k(rise))`

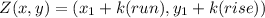
Filling that in:

`Z(x,y)=(9+(5)/(8)(-15),2+(5)/(8)(0))`

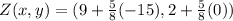
which simplifies to

`Z(x,y)=(9-(75)/(8),2+0)`

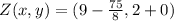
which gives us the final coordinates of Z to be
`Z(x,y)=(-(3)/(8),2)`