Question

You and a friend are stunt pilots, performing in an air show. The path of your airplane is modeled by the

equation y = −6x − 5, and your friend’s path is modeled by the equation
y = −4x^2 − 13x − 12. Do your paths cross each other? If so, then what are the coordinates of the point(s) where the paths meet?

Answer 1

The paths do not cross each other.

Step-by-step explanation

The equation that models one of the paths is

`y = - 6x - 5`

and the other is modeled by:

`y = - 4 {x}^(2) - 13x - 12`

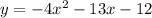
We equate the two equations and solve for their point of intersection.

`- 4 {x}^(2) - 13x - 12 = - 6x - 5`

`- 4 {x}^(2) - 13x + 6x- 12 + 5 =0`

`- 4 {x}^(2) - 7x- 7 =0`

`4 {x}^(2) + 7x + 7 =0`

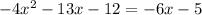
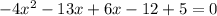
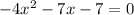
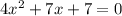
The discriminant is

`D= {b}^(2) - 4ac`

`D= {7}^(2) - 4(4)(7) = - 63`

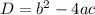
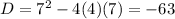
The discriminant is negative so the equation has no real roots.

This means that, the two paths do not cross.