Question

The volume of a rectangular prism is 3 25/36 cubic units, and the base area of the prism is 3 1/6 square units. The height of the rectangular prism is a. 1 1/6units b. 1 1/2 units c. 3 1/6 units d. 3 1/3 units. The number of cubic blocks, each with a volume of 1/36 cubic units, needed to fill the rectangular prism is a. 7 b. 19 c. 133 d. 152. ?

Answer 1

1st one is 1 1/6

2nd one is 133

I did the test on Plato and this was right :)

Answer 2

The height of the prism is
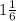`1(1)/(6)` units ⇒ answer a

The number of blocks needed is 133 ⇒ answer c

Explanation:

* Lets explain how to solve the problem

- The volume of a rectangular prism is

→ V = Area of the base × its height

- The volume of the rectangular prism is
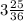`3(25)/(36)` units³

- The area of its base is
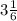`3(1)/(6)` units²

- Substitute the values of the volume and area of the base in the rule

∴
`3(25)/(36)=3(1)/(6)*h`

- Divide the two sides by
`3(1)/(6)`

∴
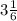
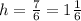`h=(7)/(6)=1(1)/(6)` units

* The height of the prism is
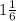`1(1)/(6)` units

- The volume of each cubic block is
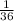`(1)/(36)` units³

- The number of blocks = volume of the prism ÷ volume of the block

∵ The volume of the prism is
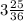`3(25)/(36)` units³

∵ The volume of each block is
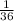`(1)/(36)` units³

∴ The number of blocks =
`3(25)/(36)` ÷
`(1)/(36)=133`

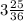∴ The number of blocks = 133

* The number of blocks needed is 133