Answer:
Part A). In 20 hr 24 minutes earth rotate 310°.
Part B). In 19 hr 6 minutes earth rotate 5 radians
Part C). 2072.4 miles point on equator rotates in 2 hours.
Explanation:
Degree that earth rotate in 24 hour = 360°
Number of radian that earth rotate in 24 hour = 2π radian
Part A).
Time taken to rotate 360° = 24 hours
Time taken to rotate 1° =
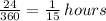
Time taken to rotate 310° =
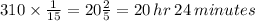
Part B).
Time taken to rotate 2π radian = 24 hours
Time taken to rotate 1 radian =

Time taken to rotate 5 radian =
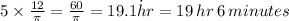
Part C).
Diameter of Earth = 7920 miles
Radius of earth, r = 3960 miles
Degree of rotation in 1 hours =

Degree of rotation in 2 hours , [tex\theta[/tex] = 15 × 2 = 30°
Length of the arc for angle 30° of circle with radius 3960 miles = Distance covered by point in 2 hours.
Length of the arc =
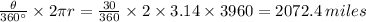
Therefore, Part A). In 20 hr 24 minutes earth rotate 310°.
Part B). In 19 hr 6 minutes earth rotate 5 radians
Part C). 2072.4 miles point on equator rotates in 2 hours.