Question

Write the equation (x - 1) ^2 + (y + 2)^ 2 = 5 in general form.

Answer 1

Hello!

The answer is:

The general form of the circle is:

`x^(2) -2x+y^(2) +4y=0`

Why?

We are given the standard form of a circle, so, to calculate the general form, we need to solve notable products.

We must remember the way to solve the notable product:

`(a+b)^(2)=a^(2)+2ab+b^(2) \\\\(a-b)^(2)=a^(2)-2ab+b^(2)`

So,

We are given the equation:

`(x-1)^(2)+(y+2)^(2)=5`

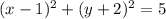
Then, solving the notable products, and adding/subtracting like terms, we have:

`x^(2) -2*1x+1+y^(2)+2*2y+4=5\\x^(2) -2x+1+y^(2) +4y+4=5\\\\x^(2) -2x+y^(2) +4y+1+4-5=0\\\\x^(2) -2x+y^(2) +4y=0`

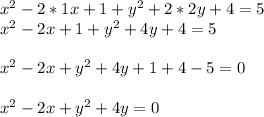
Hence, have that the general form of the circle is:

`x^(2) -2x+y^(2) +4y=0`

Have a nice day!