Hello!
The answer is:
The will paint the same fence at the same time in 2.67 hours.
Why?
From the statement we know that Shawna can paint a fence in 8 hours while Kevin can paint the same in 4 hours, and we are asked to calculate how long will it take them to paint the fence working together, so, calculating we have:
For Shawna, we have:
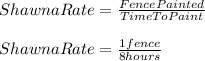
For Kevin, we have:
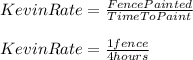
So, the combined work for both Shawna and Kevin will be:

Now, if the want to paint the same fence at the same time, we can calculate it by the following way:

Hence, the will paint the same fence at the same time in 2.67 hours.
Have a nice day!