Answer:
The product of two rational numbers is a rational number
Explanation:
I'll quickly recap the proof: a rational number is, by definition, the ratio between two integers. So, there exists four integers m,n,p,q such that
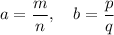
If we multiply the fractions, we have
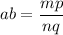
Now, mp and nq are multiplication of integers, and thus they are integers themselves. So, ab is also a ratio between integer, and thus rational.