Question

Is the inverse a function

Answer 1

Is not a function

Explanation:

A relation is a function if each value of the input set (domain) is assigned only one value of the output set (range)

Given a function
`f(x)`, the inverse of f denoted
`f ^ {- 1}(x)` is a function only if f(x) is a one-to-one function. This means that there are not in the domain of
`f(x)` two distinct values of x that produce the same value of y.

In the graph of f(x) you can see that the function is not one-to-one. Since
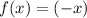`f(x) = (-x)` for all x.

For example:

`f(1) = f (-1)\\\\f (2) = f (-2)\\\\`

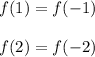
Observe the attached graph

In general, the inverse of a quadratic function f(x) is not a function