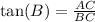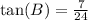Answer:

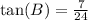
Explanation:
We have been given a right triangle. We are asked to find the
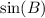 and
and
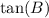 for our given triangle.
for our given triangle.
We know that sine relates opposite side of right triangle to its hypotenuse.

We can see that AC is opposite side to angle B and AB is hypotenuse of the given triangle.


We know that tangent relates opposite side of right triangle to its adjacent.
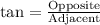
We can see that AC is opposite side to angle B and BC is adjacent side of the angle B.