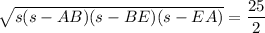I'm sure there's an easier way to do this, but this method does work:
First, AB = CD = CG + GF + FD, so FG = 2.
By the Pythagorean theorem, in triangle AFD we get
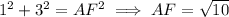
and in triangle BCG,

Angles AFD and EFG form a vertical pair, so they are congruent and have measure
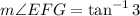
Similarly, angles BGC and FGE are congruent and have measure

Then the remaining angle in triangle EFG has measure
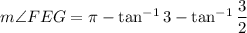
We can solve for the lengths of FE and GE exactly by applying the law of sines:
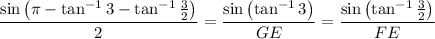

Let
 be the semiperimeter of triangle ABE, so that
be the semiperimeter of triangle ABE, so that

Then according to Heron's formula, the area of triangle ABE is