Question

What is the domain of the function f(x) = x+1 / x2-6x+8

Answer 1

is d on edge

Answer 2

For this case we have that by definition, the domain of a function, is given for all the values for which the function is defined.

We have:

`f (x) = \frac {x + 1} {x ^ 2-6x + 8}`

The given function is not defined when the denominator is equal to zero. That is to say:

`x ^ 2-6x + 8 = 0`

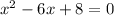
To find the roots we factor, we look for two numbers that when multiplied give as a result "8" and when added as a result "-6". These numbers are:

`-4-2 = -6\\-4 * -2 = 8`

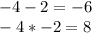
Thus, the factored polynomial is:

`(x-4) (x-2) = 0`

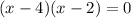
That is to say:

`x_ {1} = 4\\x_ {2} = 2`

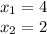
Makes the denominator of the function 0.

Then the domain is given by:

All real numbers, except 2 and 4.

Answer:

x |x≠2,4