Answer:
The current at t = 0 is
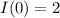 units of current
units of current
Explanation:
If the current flowing through an electric circuit is defined as the derivative of the charge as a function of time and we have the equation of the charge as a function of time, then, to find the equation of the current, we derive the equation of the charge.
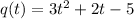

Simplifying the expression we have:
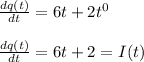
Finally, the equation that defines the current of this circuit as a function of time is:

Now to find the current at t = 0 we make


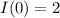 units of current
units of current