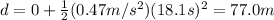Question

A disk with mass m = 9.5 kg and radius R = 0.3 m begins at rest and accelerates uniformly for t = 18.1 s, to a final angular speed of ω = 28 rad/s. 9) What is the angular acceleration of the disk? rad/s2 10) What is the angular displacement over the 18.1 s? rad 11) What is the moment of inertia of the disk? kg-m2 12) What is the change in rotational energy of the disk? J 13) What is the tangential component of the acceleration of a point on the rim of the disk when the disk has accelerated to half its final angular speed? m/s2 14) What is the magnitude of the radial component of the acceleration of a point on the rim of the disk when the disk has accelerated to half its final angular speed? m/s2 15) What is the final speed of a point on the disk half-way between the center of the disk and the rim? m/s 16) What is the total distance a point on the rim of the disk travels during the 18.1 seconds? m

Answer 1

9) 1.55 rad/s^2

The angular acceleration of the disk is given by

`\alpha = (\omega_f - \omega_i)/(t)`

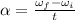
where

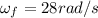`\omega_f = 28 rad/s` is the final angular speed

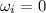`\omega_i=0` is the initial angular speed (the disk starts from rest)

t = 18.1 s is the time interval

Substituting into the equation, we find:

`\alpha = (28.1 rad/s - 0)/(18.1 s)=1.55 rad/s^2`

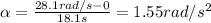
10) 253.9 rad

The angular displacement of the disk during this time interval is given by the equation:

`\theta = \omega_i t + (1)/(2)\alpha t^2`

where

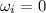`\omega_i=0` is the initial angular speed (the disk starts from rest)

t = 18.1 s is the time interval

`\alpha=1.55 rad/s^2` is the angular acceleration

Substituting into the equation, we find:

`\theta = 0 + (1)/(2)(1.55 rad/s^2)(18.1 s)^2=253.9 rad`

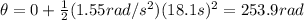
11)
`0.428 kg m^2`

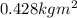
The moment of inertia of a disk rotating about its axis is given by

`I=(1)/(2)mR^2`

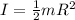
where in this case we have

m = 9.5 kg is the mass of the disk

R = 0.3 m is the radius of the disk

Substituting numbers into the equation, we find

`I=(1)/(2)(9.5 kg)(0.3 m)^2=0.428 kg m^2`

12) 167.8 J

The rotational energy of the disk is given by

`E_R = (1)/(2)I\omega^2`

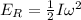
where

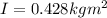`I=0.428 kg m^2` is the moment of inertia

`\omega` is the angular speed

At the beginning,
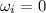`\omega_i = 0`, so the rotational energy is

`E_i = (1)/(2)(0.428 kg m^2)(0)^2 = 0`

While at the end, the angular speed is
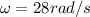`\omega=28 rad/s`, so the rotational energy is

`E_f = (1)/(2)(0.428 kg m^2)(28 rad/s)^2=167.8 J`

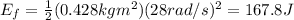
So, the change in rotational energy of the disk is

`\Delta E= E_f - E_i = 167.8 J - 0 = 167.8 J`

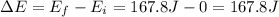
13)
`0.47 m/s^2`

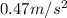
The tangential acceleration can be found by using

`a_t = \alpha r`

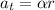
where

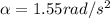`\alpha = 1.55 rad/s^2` is the angular acceleration

r is the distance of the point from the centre of the disk; since the point is on the rim,

r = R = 0.3 m

So the tangential acceleration is

`a_t = (1.55 rad/s^2)(0.3 m)=0.47 m/s^2`

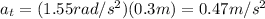
14)
`58.8 m/s^2`

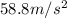
The radial (centripetal acceleration) is given by

`a_r = \omega^2 r`

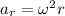
where

`\omega` is the angular speed, which is half of its final value, so

`\omega=(28 rad/s)/(2)=14 rad/s`

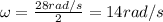
r is the distance of the point from the centre (as before, r = R = 0.3 m)

Substituting numbers into the equation,

`a_r = (14 rad/s)^2 (0.3 m)=58.8 m/s^2`

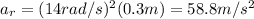
15) 4.2 m/s

The tangential speed is given by:

`v=\omega r`

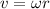
where

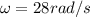`\omega = 28 rad/s` is the angular speed

r is the distance of the point from the centre of the disk, so since the point is half-way between the centre of the disk and the rim,

`r=(R)/(2)=(0.3 m)/(2)=0.15 m`

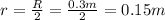
So the tangential speed is

`v=(28 rad/s)(0.15 m)=4.2 m/s`

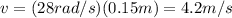
16) 77.0 m

The total distance travelled by a point on the rim of the disk is

`d=ut + (1)/(2)a_t t^2`

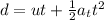
where

u = 0 is the initial tangential speed

t = 18.1 s is the time

`a_t = 0.47 m/s^2` is the tangential acceleration

Substituting into the equation, we find

`d=0+(1)/(2)(0.47 m/s^2)(18.1 s)^2=77.0 m`