Question

Coherent red light of wavelength λ = 700 nm is incident on two very narrow slits. The light has the same phase at both slits. (a) What is the angular separation in radians between the central maximum in intensity and an adjacent maximum if the slits are 0.025 mm apart? (b) What would be the separation between maxima in intensity on a screen located 1 m from the slits? (c) What would the angular separation be if the slits were 2.5 mm apart? (d) What would the separation between maxima be on a screen 25 mm from the slits? The answer is relevant to the maximum resolution along your retina that would be useful given the size of your pupil. The spacing between cones on the retina is about 10 µm. (e) How would the location of the maxima change if one slit was covered by a thin film with higher index of refraction that shifted the phase of light leaving the slit by π? Would the separation change?

Answer 1

(a) 0.028 rad

The angular separation of the nth-maximum from the central maximum in a diffraction from two slits is given by

`d sin \theta = n \lambda`

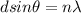
where

d is the distance between the two slits

`\theta` is the angular separation

n is the order of the maximum

`\lambda` is the wavelength

In this problem,

`\lambda=700 nm=7\cdot 10^(-7) m`

`d=0.025 mm=2.5\cdot 10^(-5) m`

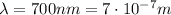
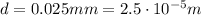
The maximum adjacent to the central maximum is the one with n=1, so substituting into the formula we find

`sin \theta = (n \lambda)/(d)=((1)(7\cdot 10^(-7) m))/(2.5\cdot 10^(-5) m)=0.028`

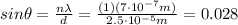
So the angular separation in radians is

`\theta= sin^(-1) (0.028) = 0.028 rad`

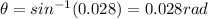
(b) 0.028 m

The screen is located 1 m from the slits:

D = 1 m

The distance of the screen from the slits, D, and the separation between the two adjacent maxima on the screen (let's call it y) form a right triangle, so we can write the following relationship:

`(y)/(D)=tan \theta`

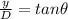
And so we can find y:

`y=D tan \theta = (1 m) tan (0.028 rad)=0.028 m`

(c)
`2.8\cdot 10^(-4) rad`

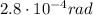
In this case, we can apply again the formula used in part a), but this time the separation between the slits is

`d=2.5 mm = 0.0025 m`

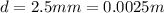
so we find

`sin \theta = (n \lambda)/(d)=((1)(7\cdot 10^(-7) m))/(0.0025 m)=2.8\cdot 10^(-4)`

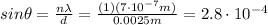
And so we find

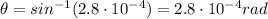
`\theta= sin^(-1) (2.8\cdot 10^(-4)) = 2.8\cdot 10^(-4) rad`

(d)
`7.0\cdot 10^(-6) m = 7.0 \mu m`

This part can be solved exactly as part b), but this time the distance of the screen from the slits is

`D=25 mm=0.025 m`

So we find

`y=D tan \theta = (0.025 m) tan (2.8\cdot 10^(-4) rad)=7.0\cdot 10^(-6) m = 7.0 \mu m`

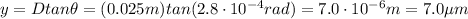
(e) The maxima will be shifted, but the separation would remain the same

In this situation, the waves emitted by one of the slits are shifted by
`\pi` (which corresponds to half a cycle, so half wavelength) with respect to the waves emitted by the other slit.

This means that the points where previously there was constructive interference (the maxima on the screen) will now be points of destructive interference (dark fringes); on the contrary, the points where there was destructive interference before (dark fringes) will now be points of maxima (bright fringes). Therefore, all the maxima will be shifted.

However, the separation between two adjacent maxima will not change. In fact, tall the maxima will change location exactly by the same amount; therefore, their relative distance will remain the same.