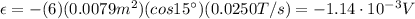Answer:
Step-by-step explanation:
The induced emf in the loop is given by Faraday's Newmann Lenz law:
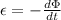 (1)
(1)
where
 is the variation of magnetic flux
is the variation of magnetic flux
 is the variation of time
is the variation of time
The magnetic flux through the coil is given by
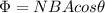 (2)
(2)
where
N = 6 is the number of loops
A is the area of each loop
B is the magnetic field strength
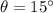 is the angle between the direction of the magnetic field and the normal to the area of the coil
is the angle between the direction of the magnetic field and the normal to the area of the coil
Since the radius of each loop is r = 5.00 cm = 0.05 m, the area is
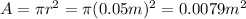
Substituting (2) into (1), we find
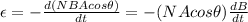
where
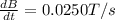 is the rate of variation of the magnetic field
is the rate of variation of the magnetic field
Substituting numbers into the last formula, we find