Question

Solve the following equation for W.
P=2L+2W

Answer 1

**Disclaimer** Hi there! I assumed the question is to represent W in terms of all other variables (P, L). The following answer corresponds to this understanding. If it is incorrect, please let me know and I will modify my answer.

Answer: W = (P/2) - L

Explanation:

Given equation

P = 2L + 2W

Factorize 2 out

P = 2 (L + W)

Divide 2 on both sides

P / 2 = 2 (L + W) / 2

P / 2 = L + W

Subtract L on both sides

(P / 2) - L = L + W - L

`\Large\boxed{W=(P)/(2) -L}`

Hope this helps!! :)

Please let me know if you have any questions

Answer 2

`\displaystyle{W = (P)/(2) - L}`

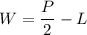
Explanation:

To solve for W, we have to isolate the W-variable. First, we can factor the expression 2L + 2W to 2(L+W):

`\displaystyle{P = 2(L+W)}`

Next, we'll be dividing both sides by 2:

`\displaystyle{(P)/(2) = (2(L+W))/(2)}\\\\\displaystyle{(P)/(2) = L+W}`

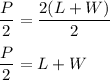
Then subtract both sides by L:

`\displaystyle{(P)/(2) - L= L+W-L}\\\\\displaystyle{(P)/(2) - L= W}`

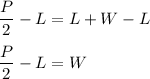
Therefore, we'll obtain W = P/2 - L.

Note that the given formula is perimeter formula of a rectangle where Perimeter = 2 * Length + 2 * Width.

So if we solve for W (Width) then we'll get Width = Perimeter / 2 - Length which can be useful to find width with given perimeter and length.