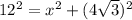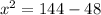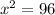Answer:
Part 1)
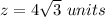
Part 2)
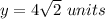
Part 3)

Explanation:
see the attached figure with letters to better understand the problem
step 1
Find the value of z
In the right triangle DBC
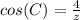 ----> equation A
----> equation A
In the right triangle ABC
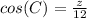 ----> equation B
----> equation B
equate equation A and equation B

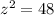
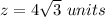
step 2
In the right triangle DBC
Applying the Pythagoras Theorem

we have
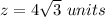
substitute
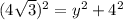
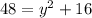
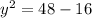
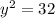
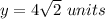
step 3
Find the value of x
In the right triangle ABC
Applying the Pythagoras Theorem
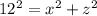
we have
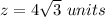
substitute