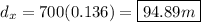Hi there!
We can begin by solving for the time taken for the bullet to travel a VERTICAL distance of 0.09 m due to the effects of gravity.
We can use the kinematic equation for uniform acceleration:

Since there is no initial vertical velocity:
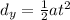
Rearrange to solve for time. (a = g = 9.8 m/s²)
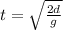
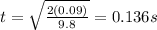
Now, we can use the distance, speed, and time equation in the horizontal direction:

Plug in the values: