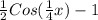Answer:
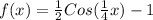
Explanation:
Consider the function f(x) = cos x. Noted below are the points of transformations of the cos function
- The function f(x) =
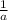 cos x is a vertical compression of the original by a factor of a
cos x is a vertical compression of the original by a factor of a - The function f(x) = cos
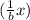 is a horizontal stretch of the original function by a factor b
is a horizontal stretch of the original function by a factor b - The function f(x) = cosx - c is the original function shifted down c units
Considering the points above, we can now write down the "transformed" cosine function's equation:
f(x) =