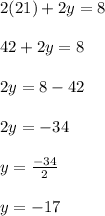Answer:
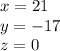
Explanation:
Multiply the second equation by -1:
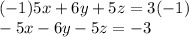
Add this equation and the third equation and solve for "z":
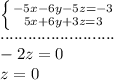
Substitute
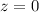 into two original equations:
into two original equations:
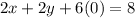
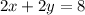 [Equation A]
[Equation A]
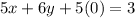
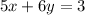 [Equation B]
[Equation B]
Multiply the Equation A by -3, add both equations and then solve for "x":
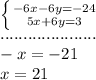
Substitute
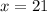 into the Equation A or the Equation B and solve for "y":
into the Equation A or the Equation B and solve for "y":