Answer:
The value of x is 1.46.
Explanation:
Given : Equation
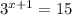
To find : Solve for x using the change of base formula log base b of y equals log y over log b ?
Solution :
Equation
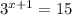
Applying the logarithmic property,
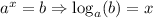
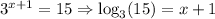
Applying change base formula in LHS,
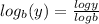
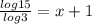


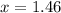
Therefore, the value of x is 1.46.