Question

What is the equation of the tangent line passing through the point (0, 3) of the graph of the function f(x) = x2 − 2x + 3?

Answer 1

Answer: y = -2x + 3

Step-by-step explanation: plato

Answer 2

y = -2x + 3

Explanation:

The given equation is:

`f(x)=x^2-2x+3`

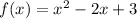
First we need to find the slope of the tangent line. This can be done by finding the derivative of the given function.

`f'(x)=2x-2`

Slope of the tangent will be the value of the derivative at the given point. So the slope of tangent is:

`f'(0)=2(0)-2=-2`

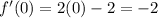
Now we have slope of the tangent line and a point (0, 3) on the tangent. The point (0,3) is the y-intercept of the tangent line. So we can use slope-intercept form to directly write the equation of the line.

The slope intercept form of an equation is:

y = mx + c

where m is the slope and c is the y-intercept.

Using the values: m = -2 and c = 3, we get:

y = -2x + 3

This equation represents the tangent line