Question

The equation of a circle is x^2 + y^2 -4x + 2y -11 =0 . What are the center and the radius of the circle? Show your work.

Answer 1

Center: (2,-1)

Radius: 4 units

Explanation:

Equation of the circle in standard form is:

`x^(2)+y^(2)+2gx+2fy+c=0`

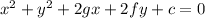
The radius of this circle is located at (-g, -f) and its radius is equal to:

`r=\sqrt{g^(2)+f^(2)-c}`

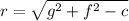
The given equation of circle is:

`x^(2)+y^(2)-4x+2y-11=0`

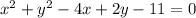
Re-writing this equation in a form similar to the standard equation:

`x^(2)+y^(2)+2(-2)x+2(1)y-11=0`

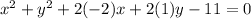
Comparing this equation with standard equation we can say:

g= -2

f = 1

c = -11

So, the center of the circle will be located at (-g, -f) = (2, -1)

And the radius will be =
`\sqrt{g^(2)+f^(2)-c} =\sqrt{(-2)^(2)+(1)^(2)-(-11)} =√(16) =4`

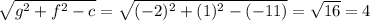
Thus the radius of the given circle is 4 units.