Question

The point (-3, 7) is on the terminal side of angle Θ, in standard position. What are the values of sine, cosine, and tangent of Θ?

I need an answer ASAP!!! Thank You!!

Answer 1

sinθ = 0.9191; cosθ = -0.3939; tanθ = -2.333

Explanation:

The point (-3, 7) is in the second quadrant, so θ is an obtuse angle as in the figure below.

AB = 7

OA = -3

According to Pythagoras,

OB² = OA² + AB² = (-3)² + 7² = 9 + 49 = 58

OB = √58 = 7.616

`\begin{array}{rcccccr}\sin\theta & = & (AB )/(OB) & = &(7 )/(7.616 ) & = & 0.9191\\\\\cos\theta & = & (OA )/(OB) & = & (-3 )/(7.616 ) & = & -0.3939 \\\\\tan\theta & = & (AB )/(OA) & = & (7 )/(-3 ) & = & -2.333 \\\end{array}\\`

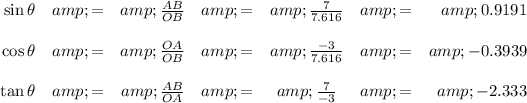
sinθ = 0.9191; cosθ = -0.3939; tanθ = -2.333