Question

Which polynomial can be simplified to a difference of squares

Answer 1

I am assuming your doing edgunity like me if thats the case the correct answer is b

Explanation:

i know this because thats a polynomial that can simplified into squares

Answer 2

Hello!

The answer is:

The polynomial that can be simplified to a difference of squares is the second polynomial:

`16a^(2)-4a+4a-1=16a^(2)=(4a)^(2)-(1)^(2)=(4-1)(4+1)`

Why?

To solve this problem, we need to look for which of the given quadratic terms given for the different polynomials can be a result of squaring (elevating by two).

So,

Discarding, we have:

The quadratic terms of the given polynomials are:

`First=10a^(2)`

`Second=16a^(2)`

`Third=25a^(2)`

`Fourth=24a^(2)`

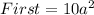
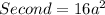
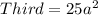
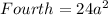
We have that the coefficients of the quadratic terms that can be obtained by squaring are:

`16a^(2) =(4a)^(2) \\\\25a^(2) =(5a)^(2)`

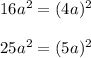
The other two coefficients are not perfect squares since they can not be obtained by square rooting whole numbers.

So, the first and the fourth polynomial are discarded and cannot be simplified to a difference of squares at least using whole numbers.

Therefore, we need to work with the second and the third polynomial.

For the second polynomial, we have:

`16a^(2) -4a+4a-1=16a^(2)=(4a)^(2)-(1)^(2) =(4-1)(4+1)`

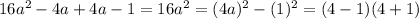
So, the second polynomial can be simplified to a difference of squares.

For the third polynomial, we have:

`25a^(2) +6a-6a+36=16a^(2)+36=(5a)^(2)+(6)^(2)`

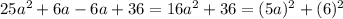
So, the third polynomial cannot be simplified to a difference of squares since it's a sum of squares.

Hence, the polynomial that can be simplified to a difference of squares is the second polynomial:

`16a^(2)-4a+4a-1=16a^(2)=(4a)^(2)-(1)^(2)`