Hello!
The answer is:
The point C. (2,6) is a solution to the system of inequalities.
Why?
To check what point is a solution to the system of inequalities, the point must satisfy both inequalities, so:
We are given the inequalities:
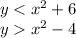
Checking, we have:
- Substituting A(0,8), we have:
First inequality,
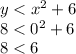
Now, since 8 is not less than 6, we know that A (0,8) is not a solution to the system of inequalities since it does not satisfy both inequalities.
- Substituting B(4,2), we have:
First inequality,
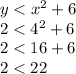
Second inequality,
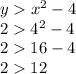
Now, since 2 is not greater than 12, we know that B(4,2) is not a solution to the system of inequalities since it does not satisfy both inequalities.
- Substituting C(2,6), we have:
First inequality,
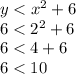
Second inequality,
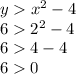
Now, as we can se, both inequalities are satisfied since 6 is less than 10 and greater than 0, so C(2,6) is a solution to the system.
- Substituting D(-2,-4)
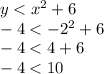
Second inequality,
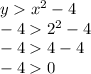
Now, since -4 is not greater than 0, we know that D(-2,-4) is not a solution to the system of inequalities since it does not satisfy both inequalities.
Hence,
Only the point C. (2,6) is a solution to the system of inequalities.
Have a nice day!