Question

Suppose that the value of a stock varies each day from $10.82 to $25.17 with a uniform distribution. Find the third quartile, i.e., 75% of all days the stock is below what value

Answer 1

Answer: Third quartile =$21.58

Explanation:

Let x be value of a stock .

For uniform distribution,

probability density function =
`(1)/(b-a)=(1)/(25.17-10.82)=\frac1{14.35}=(100)/(1435)`

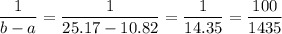
Let a be the stock value such that P(x<a) =75% or 0.75

`\Rightarrow\ \int ^(a)_(10.82)f(x)\ dx=0.75\\\\\Rightarrow\ \int ^(a)_(10.82)(100)/(1435)\ dx=0.75\\\\\Rightarrow(100)/(1435)[x]^(a)_(10.82)=0.75\\\\\Rightarrow (100)/(1435)(a-10.82)=0.75\\\\\Rightarrow a-10.82=0.75*(1435)/(100)\\\\\Rightarrow a-10.82=10.7625\\\\\Rightarrow a= 10.7625+10.82= $$21.5825\approx $$21.58`

Hence, 75% of all days the stock is below $21.58 or Third quartile =$21.58 .