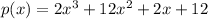Question

I am so confused and im really struggling and this is the only question i need ​

Answer 1

A polynomial of degree 3 has exactly 3 solutions if we use complex numbers. If the polynomial has real coefficients and z is a complex solution, i.e.,
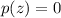`p(z)=0`, the conjugate is also a solution:
`p(\overline{z})=0`

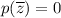
So, in the first case, if we know that 1-i is a solution, the conjugate 1+i must be a solution as well.

So, we know all the roots: -5, 1+i, 1-i.

When you know the solutions
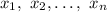`x_1,\ x_2,\ldots,\ x_n` of a polynomial, you can write the polynomial (up to multiples) as

`p=(x-x_1)(x-x_2)\ldots(x-x_n)`

So, in your case, we have

`p(x) = (x+5)(x-1-i)(x-1+i) = x^3+3x^2-8x+10`

You can check that indeed
`p(0)=10`, because the constant term is 10, so, we're ok with this

The second exercise is exactly the same: the solutions -6, i, -i identify the polynomial

`p(x)=(x+6)(x-i)(x+i)=x^3+6x^2+x+6`

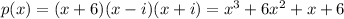
In this case, P(-3)=30, so if we want P(-3)=60 we have to multiply everything by 2:

`p(x)=2x^3+12x^2+2x+12`