Question

The table below shows the function f.

x: 2,3,4,5,6

f(x) ?, 15, 23, 39, 71

Determine the value of f(2) that will lead to an average rate of change of 15 over the interval [2, 6].

A. 11
B.-1
C.9
D. 41

Answer 1

Answer: The correct option is (A) 11.

Step-by-step explanation: Given the following table that shows the function f :

x 2 3 4 5 6

f(x) ? 15 23 39 71

We are to determine the value of f(2) that will lead to an average rate of change of 15 over the interval [2, 6].

We know that

the rate of change of a function g(x) over an interval [a, b] is given by

`R=(g(b)-g(a))/(b-a).`

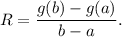
From the table, we note that

f(6) = 71 and f(2) = ?

So, the rate of change of the function f(x) over the interval [2, 6] is given by

`R=(f(6)-f(2))/(6-2)\\\\\\\Rightarrow 15=(72-f(2))/(4)\\\\\Rightarrow 15*4=72-f(2)\\\\\Rightarrow 60=71-f(2)\\\\\Rightarrow f(2)=71-60\\\\\Rightarrow f(2)=11.`

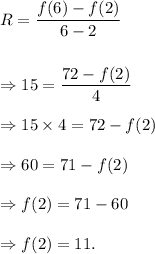
Thus, the required value of f(2) is 11.

Option (A) is CORRECT.

Answer 2

A. 11

Explanation:

The average rate of change of the given function over the interval [2,6] is simply the slope of the secant line connecting the point (2,f(2)) and (6,f(6)).

This implies that, the average rate of change over [2,6]

`=(f(6)-f(2))/(6-2)`

`=(f(6)-f(2))/(4)`

From the table f(6)=71

Since we want the average rate of change to be 15, we have;

`15=(71-f(2))/(4)`

This implies that;

`4*15=71-f(2)`

`60=71-f(2)`

`60-71=-f(2)`

`-11=-f(2)`

`11=f(2)`

The correct choice is A.