Answer:
The graph of g(x) is shifted horizontally 5 units to the right of the graph of f(x).
Explanation:
Given :The function f(x)=
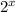 and g(x)=f(x+k). If k= -5,
and g(x)=f(x+k). If k= -5,
To find : what can be concluded about the graph of g(x).
Solution : The function parent f(x) =
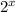 .
.
By the Transformation rule if f(x) transformed to f(x+k) it would shifted horizontally toward left by k units .
Then ,
g(x)=f(x+ (-5)) would be b. 5 units to the right of the graph of f(x).
Therefore, The graph of g(x) is shifted horizontally 5 units to the right of the graph of f(x).