Question

Identify each function that has a remainder of -3 when divided x+6

A) x^5 + 2x^2 - 30x + 30
B) x^4 + 4x^3 - 21x^2 - 53x + 12
C) x^3 - 10x^2 - 7
D) x^4 + 6x^3 - 10x - 63

Answer 1

D

Explanation:

According to remainder theorem, you can know the remainder of these polynomials if you plug in x = -6 into them.

So we will plug in -6 into x of all the polynomials ( A through D) and see which one equals -3.

For A:

`x^5 + 2x^2 - 30x + 30\\=(-6)^5 + 2(-6)^2 - 30(-6) + 30\\=-7494`

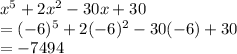
For B:

`x^4 + 4x^3 - 21x^2 - 53x + 12\\=(-6)^4 + 4(-6)^3 - 21(-6)^2 - 53(-6) + 12\\=6`

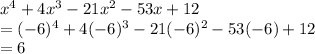
For C:

`x^3 - 10x^2 - 7\\=(-6)^3 - 10(-6)^2 - 7\\=-583`

For D:

`x^4 + 6x^3 - 10x - 63\\=(-6)^4 + 6(-6)^3 - 10(-6) - 63\\=-3`

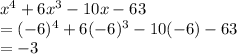
The only function that has a remainder of -3 when divided by x + 6 is the fourth one, answer choice D.