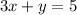Answer:
See Below.
Explanation:
We are given a point P(x, y). It is equidistant from A(3, 6) and B(-3, 4).
So, let's first determine the distance from P to each point. We can use the distance formula:
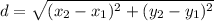
Segment PA:
We will let P(x, y) be (x₂, y₂) and A(3, 6) be (x₁, y₁). By substitution:
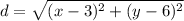
This represents the distance from P to A.
Segment PB:
Again, we will let P(x, y) be (x₂, y₂) and B(-3, 4) be (x₁, y₁). By the distance formula:
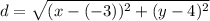
We may simplify:
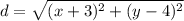
Now, we know that the two distances are equivalent. Hence:

Simplify. Square both sides:
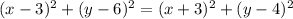
Square:
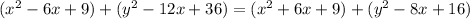
Subtract all terms from the right:
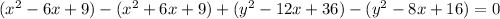
Distribute the negative:
![[(x^2-6x+9)+(-x^2-6x-9)]+[(y^2-12y+36)+(-y^2+8y-16)]=0](https://img.qammunity.org/2022/formulas/mathematics/high-school/a5x5mrczoqmj17nvc3pm49y57zg9bqehsq.png)
Rearrange:
![[(x^2-x^2)+(-6x-6x)+(9-9)]+[(y^2-y^2)+(-12y+8y)+(36-16)]=0](https://img.qammunity.org/2022/formulas/mathematics/high-school/86gyameco2l2c2cnc4jdxpx70suhck5xi1.png)
Combine like terms;
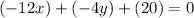
We can divide both sides by -4:

Finally, adding 5 to both sides produces: