Question

A disk rotates about its central axis starting from rest and accelerates with constant angular acceleration. At one time it is rotating at 11.0 rev/s; 50.0 revolutions later, its angular speed is 20.0 rev/s. Calculate (a) the angular acceleration (rev/s2), (b) the time required to complete the 50.0 revolutions, (c) the time required to reach the 11.0 rev/s angular speed, and (d) the number of revolutions from rest until the time the disk reaches the 11.0 rev/s angular speed. (a) Number Enter your answer in accordance to item (a) of the question statement Unit Choose the answer from the menu in accordance to item (a) of the question statement (b) Number Enter your answer in accordance to item (b) of the question statement Unit Choose the answer from the menu in accordance to item (b) of the question statement (c) Number Enter your answer in accordance to item (c) of the question statement Unit Choose the answer from the menu in accordance to item (c) of the question statement (d) Number Enter your answer in accordance to item (d) of the question statement Unit Choose the answer from the menu in accordance to item (d) of the question statement

Answer 1

(a)
`2.79 rev/s^2`

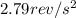
The angular acceleration can be calculated by using the following equation:

`\omega_f^2 - \omega_i^2 = 2 \alpha \theta`

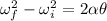
where:

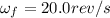`\omega_f = 20.0 rev/s` is the final angular speed

`\omega_i = 11.0 rev/s` is the initial angular speed

`\alpha` is the angular acceleration

`\theta=50.0 rev` is the number of revolutions made by the disk while accelerating

Solving the equation for
`\alpha`, we find

`\alpha=(\omega_f^2-\omega_i^2)/(2d)=((20.0 rev/s)^2-(11.0 rev/s)^2)/(2(50.0 rev))=2.79 rev/s^2`

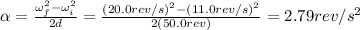
(b) 3.23 s

The time needed to complete the 50.0 revolutions can be found by using the equation:

`\alpha = (\omega_f-\omega_i)/(t)`

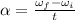
where

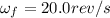`\omega_f = 20.0 rev/s` is the final angular speed

`\omega_i = 11.0 rev/s` is the initial angular speed

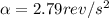`\alpha=2.79 rev/s^2` is the angular acceleration

t is the time

Solving for t, we find

`t=(\omega_f-\omega_i)/(\alpha)=(20.0 rev/s-11.0 rev/s)/(2.79 rev/s^2)=3.23 s`

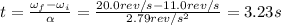
(c) 3.94 s

Assuming the disk always kept the same acceleration, then the time required to reach the 11.0 rev/s angular speed can be found again by using

`\alpha = (\omega_f-\omega_i)/(t)`

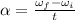
where

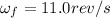`\omega_f = 11.0 rev/s` is the final angular speed

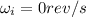`\omega_i = 0 rev/s` is the initial angular speed

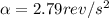`\alpha=2.79 rev/s^2` is the angular acceleration

t is the time

Solving for t, we find

`t=(\omega_f-\omega_i)/(\alpha)=(11.0 rev/s-0 rev/s)/(2.79 rev/s^2)=3.94 s`

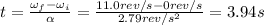
(d) 21.7 revolutions

The number of revolutions made by the disk to reach the 11.0 rev/s angular speed can be found by using

`\omega_f^2 - \omega_i^2 = 2 \alpha \theta`

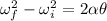
where:

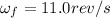`\omega_f = 11.0 rev/s` is the final angular speed

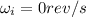`\omega_i = 0 rev/s` is the initial angular speed

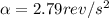`\alpha=2.79 rev/s^2` is the angular acceleration

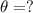`\theta=?` is the number of revolutions made by the disk while accelerating

Solving the equation for
`\theta`, we find

`\theta=(\omega_f^2-\omega_i^2)/(2\alpha)=((11.0 rev/s)^2-0^2)/(2(2.79 rev/s^2))=21.7 rev`