Answer:
16x² − 56x + 49
Explanation:
The square of difference of two terms can be expressed as a perfect square trinomial in the following manner:
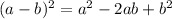
The symbol with square terms must be always positive. So this removes the first two options from the given choices. The correct option is either c or d.
Only the option d can be expressed as a perfect square as shown below:
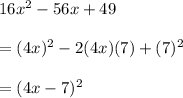
Option c would have been correct if it was -30b instead of -15b i.e. the middle term is twice the product of first and second term.
So, option D is correct