Answer:
total number of roots =4
Explanation:
the total number of roots of each polynomial function using the factored form

Given f(x) is in factored form, to get the roots we look at the factors and the exponents.
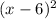 has exponent 2, so we have two roots 6 and 6
has exponent 2, so we have two roots 6 and 6
like that
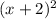 gives us two roots because it has exponent 2
gives us two roots because it has exponent 2
So total number of roots for this polynomial function is 4