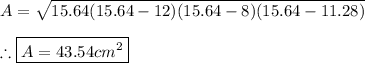Answer:
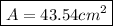
Explanation:
To find this area we will use the law of cosine and the Heron's formula. First of all, let't find the unknown side using the law of cosine:
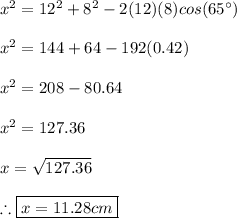
Heron's formula (also called hero's formula) is used to find the area of a triangle using the triangle's side lengths and the semiperimeter. A polygon's semiperimeter s is half its perimeter. So the area of a triangle can be found by:
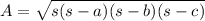 being
being
 the corresponding sides of the triangle.
the corresponding sides of the triangle.
So the semiperimeter is:
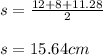
So the area is: