Question

Which point lies on a circle with a radius of 5 units and center at P(6,1)?

a. Q(1, 11)
b. R(2, 4)
c. S(4, -4)
d. T(9, -2)

Answer 1

Option B.
`R(2,4)`

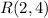
Explanation:

we know that

If a ordered pair lie on the circle. then the ordered pair must satisfy the equation of the circle

step 1

Find the equation of the circle

we know that

The equation of the circle in center radius form is equal to

`(x-h)^(2)+(y-k)^(2)=r^(2)`

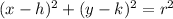
where

r is the radius of the circle

(h,k) is the center of the circle

substitute the values

`(x-6)^(2)+(y-1)^(2)=5^(2)`

`(x-6)^(2)+(y-1)^(2)=25`

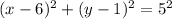
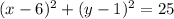
step 2

Verify each case

case A)
`Q(1, 11)`

substitute the value of
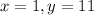`x=1, y=11` in the equation of the circle and then compare the results

`(1-6)^(2)+(11-1)^(2)=25`

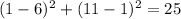
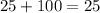`25+100=25` ------> is not true

therefore

the ordered pair Q not lie on the circle

case B)
`R(2,4)`

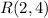
substitute the value of
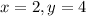`x=2, y=4` in the equation of the circle and then compare the results

`(2-6)^(2)+(4-1)^(2)=25`

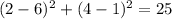
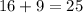`16+9=25` ------> is true

therefore

the ordered pair R lie on the circle

case C)
`S(4,-4)`

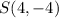
substitute the value of
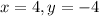`x=4, y=-4` in the equation of the circle and then compare the results

`(4-6)^(2)+(-4-1)^(2)=25`

`4+25=25` ------> is not true

therefore

the ordered pair S not lie on the circle

case D)
`T(9,-2)`

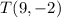
substitute the value of
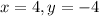`x=4, y=-4` in the equation of the circle and then compare the results

`(9-6)^(2)+(-2-1)^(2)=25`

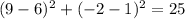
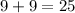`9+9=25` ------> is not true

therefore

the ordered pair T not lie on the circle