Question

Which of the following expressions are equivalent to 2/x^4-y-^4 choose all that apply

Answer 1

B and D.

Explanation:

B. The numerator = 2*1 = 2 and x^4 - y^4 = (x^2 - y^2)(x^2 + y^2).

D . (x^2)^2 - (y^2)^2 = x^4 - y^4.

Answer 2

The answer are options

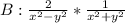`B: (2)/(x^2-y^2)*(1)/(x^2+y^2)` and

`D: (2)/((x^2)^2-(y^2)^2)`

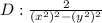
Explanation:

As all the options are multiplication of fractions the option A cannot be an answer because the numerator multiplication is 1 and different to 2. In the case of option C, observe that if we multiply the denominators we have:

`(x^2-y^2).(x^2-y^2) = (x^2-y^2)^2`

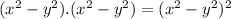
As we know for the expanding of the square binomials:

`(x^2-y^2)^2 = (x^2)^2 -2*x^2.y^2 + (y^2)^2 = x^4 +2*x^2y^2 + y^4`

Which is different from the denominator compared:

`x^4 -2*x^2.y^2+y^(4) \\eq x^4 - y^4`

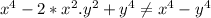
Thus option B cannot be an answer either.

Noting that the denominator compared is a square of two difference by definition, therefore, can be written as:

`x^4 - y^4 = (x^2 - y^2)(x^2 + y^2)`

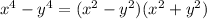
This results in the same denominator as option B. So, option B is a possible answer.

Finally, in the denominator of option D, we can solve the exponents of this factor.

`(x^(2))^(2)-(y^(2))^(2)=x^4 - y^4`

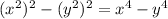
Which results in the same as the denominator compared, this let option D to be a possible answer.